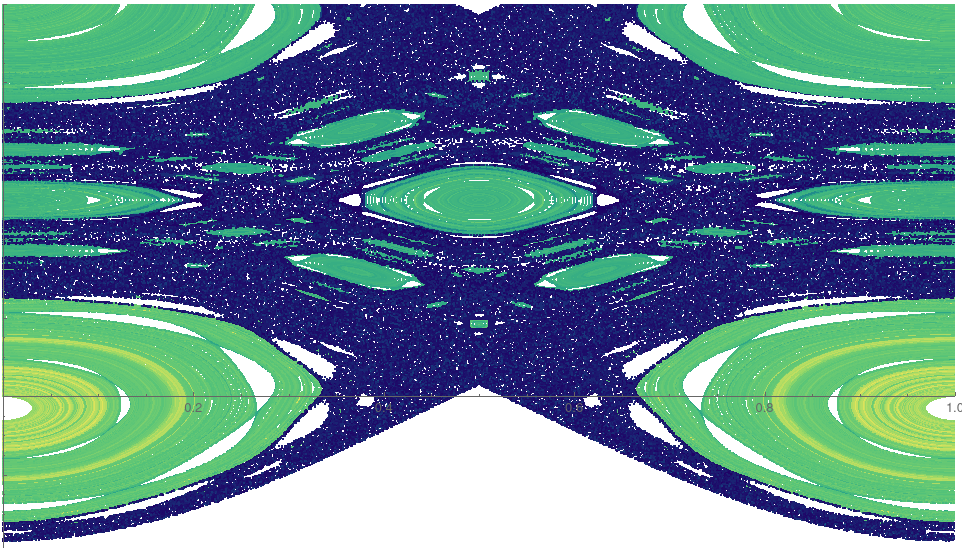
measuring integrability

In essense, integrable systems are differential equations with enough symmetry to provide an almost complete understanding of the solutions. Integrable systems are rare, yet, understanding them is crucial to the study of many non-integrable systems. Such examples are the \(n\)-body problem and the magnetic confinement of fusion.

This research investigates and develops mathematical tools for investigating how close to integrable a given system is. Two important tools for which I have helped develop are converse KAM theory and weighted Birkhoff averaging.

Converse KAM Theory

The Louiville-Arnol’d Theorem proves that all integrable systems are foliated by invariant tori, that is, every generic solution of an integrable system lies on a torus of some dimension. Komolgorov-Arnold-Moser (KAM) theory reveals that, if a system is ‘close’ to integrable then some solutions still lie on so called KAM tori, and are generically nestled in a sea of chaos. Converse KAM theory asks: how can we find when a given solution to a differential equation does not lie on a torus? By understanding how many such orbits do not lie on tori, a measure of how integrable a given system is can be formed. In (Duignan & Meiss, 2021) we review this technique in the context of magnetic confinement devices.

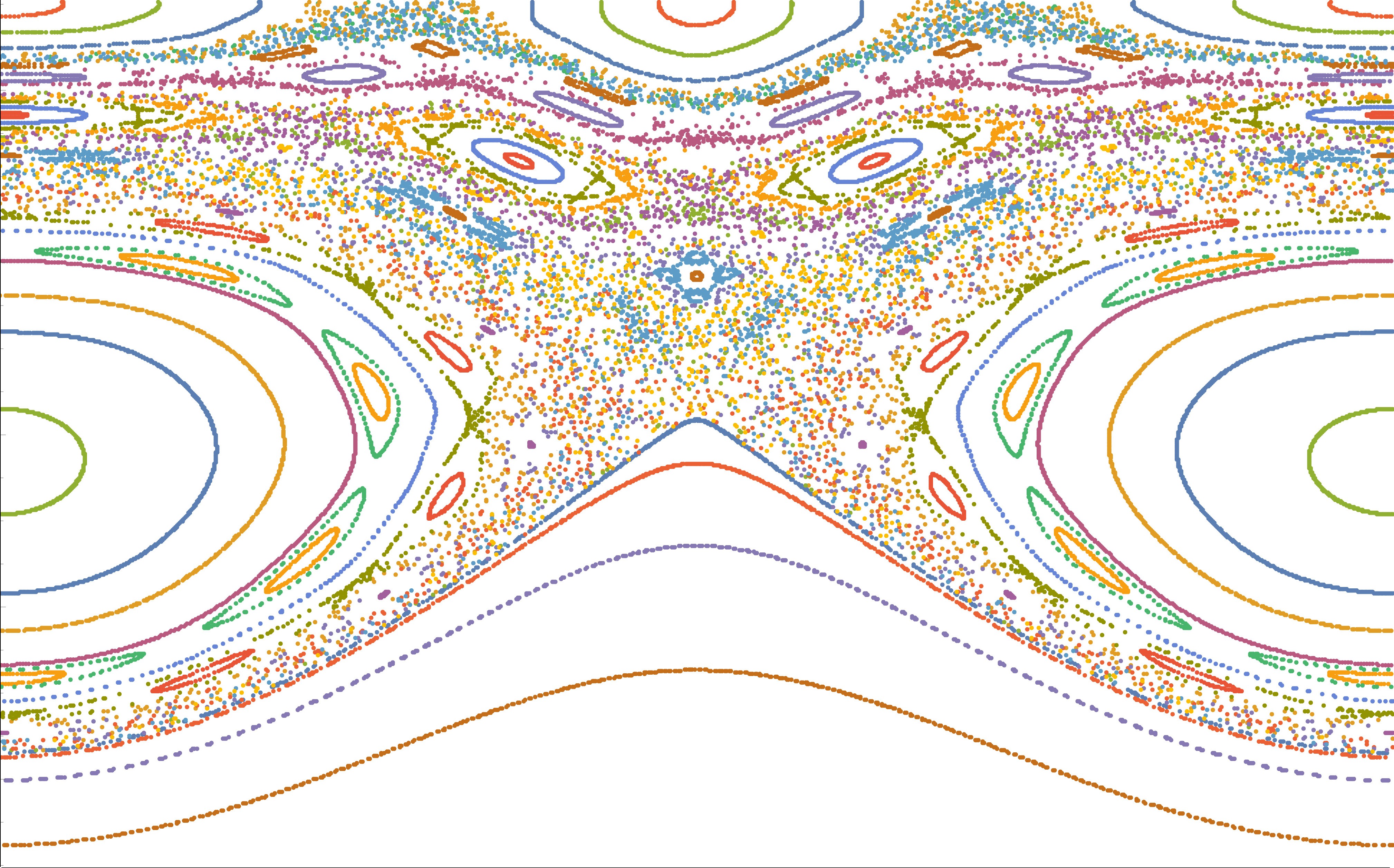
Weighted Birkhoff Averaging

A foundational theorem due to Birkhoff states for a measure preserving, ergodic flow, that the time average of a given function is equivalent to the space average of the function. It has been recently understood that there is a difference between the convergence rate for a chaotic orbit and a quasi-periodic orbit when the time average is considered with respect to a weight function which is a smooth bump function. By understanding this difference, one can use the relative rate of convergence of the average along a given orbit to discriminate between regular and chaotic motion. Ultimately, this provides a tool to evaluate how many tori may exist in a given system, and hence, how integrable it may be considered.